Ideal Poisson-Voronoi tessellations

In brief: What happens to a Poisson-Voronoi tessellation in the low intensity limit? For the Voronoi tessellation of an homogeneous Poisson process in Euclidean space, not much … However, if the underlining space has hyperbolic features (exponential volume growth) something magic may happen: contrary to the Euclidean setting, a limiting non-trivial Ideal Poisson-Voronoi tessellation (IPVT) may appear as the intensity of the Poisson point process tends to 0!

For the hyperbolic space \( \mathbb{H}_d \) of dimension \( d \), the IPVT, called \(\mathcal{V}_d \), can be constructed from a Poisson process of points in the space of horospheres in \( \mathbb{H}_d \). Thanks to Möbius invariance of \(\mathcal{V}_d \), one can focus on the cell containing the origin, called \( \mathcal{C}_d \).

Above there is a manipulatable sample of \( \mathcal{C}_3\) depicted in the Poincaré ball model (“jewel’’) —see top figure for more samples. Below, you can interact with the boundary \( \partial\mathcal{C}_3\) of the same sample in the upper half-space model of \( \mathbb{H}_3 \) (“foam’’).

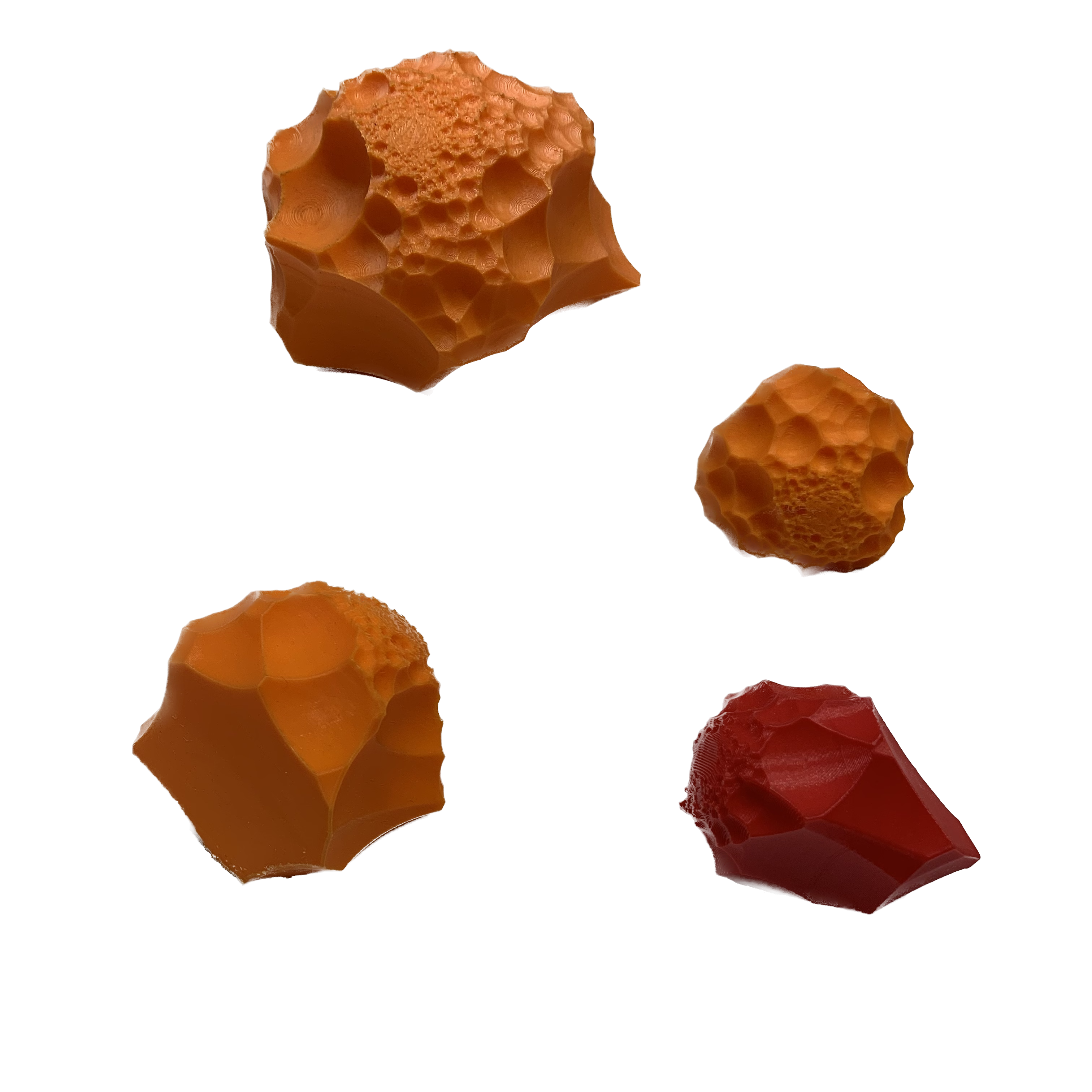
Our Poissonian description of \( \mathcal{C}_d\) even allows to easily 3D-print realizations of \( \mathcal{C}_3\) in the Poincaré ball model of \( \mathbb{H}_3 \):

Reference paper:

Ideal Poisson-Voronoi tessellations on hyperbolic spaces. Matteo D’Achille, Nicolas Curien, Nathanaël Enriquez, Russell Lyons and Meltem Ünel, 2023
arXiv preprint: 2303.16831

For further images, videos and animations on this paper see Russ Lyons’ dedicated gallery: https://rdlyons.pages.iu.edu/ipvt.html